Pedro Miraldo, Francisco Eiras, Srikumar Ramalingam

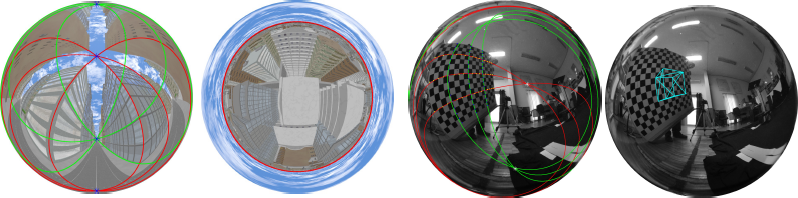
Abstract: Vanishing points and vanishing lines are classical geometrical concepts in perspective cameras that have a lineage dating back to 3 centuries. A vanishing point is a point on the image plane where parallel lines in 3D space appear to converge, whereas a vanishing line passes through 2 or more vanishing points. While such concepts are simple and intuitive in perspective cameras, their counterparts in catadioptric cameras (obtained using mirrors and lenses) are more involved. For example, lines in the 3D space map to higher degree curves in catadioptric cameras. The projection of a set of 3D parallel lines converges on a single point in perspective images, whereas they converge to more than one point in catadioptric cameras. To the best of our knowledge, we are not aware of any systematic development of analytical models for vanishing points and vanishing curves in different types of catadioptric cameras. In this paper, we derive parametric equations for vanishing points and vanishing curves using the calibration parameters, mirror shape coefficients, and direction vectors of parallel lines in 3D space. We show compelling experimental results on vanishing point estimation and absolute pose estimation for a wide range of catadioptric cameras in both simulations and real experiments.

Keywords: vanishing points, catadioptric cameras, non-cental systems.